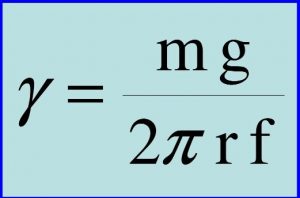If I were to redo my research question, I’d definitely strive for more trials. Since the experiment deals with very small numbers to several significant figures, clearly more precise types of equipment would be better. If possible, I’d love to try and use a stalagmometer device. However, I could see that there’s a possibility that it won’t be purchased. However, I still wanted to improve the precision and accuracy of the model of this experiment itself. The water in the syringe kept dropping in temperature, producing surface tension values for a different temperature. Hence, next time, I want to ambitiously create another model, just like a water bath, the temperature is guaranteed kept constant the whole time. It would have a tip opener coming out at the bottom so that water droplets could drop and experiments could be conducted. Then, the accuracy of the data collection could be raised tremendously.
Category: Researcher’s Reflecting Space
EE Day Progression
I predicted even before the EE day that there will be a lot of different problems arise from my EE experiment. And that’s exactly what happened today. First of all, my original method involves using a burette. However, it’s really difficult to measure the temperature of the water drops exactly. Since it’s a long narrow tube, it’s difficult to assume that the water on the top of the burette has the same temperature as the water dripping out of the burette. Then, after taking some tour to the different science department, I was casually introduced to using a shorter-length and bigger-width syringe instead. And I realise that it can solve my problems better. Now, the liquid can be mixed together equally, the top can be closed by a stopper, and the syringe can be wrapped around with aluminium foil to reduce heat loss. Hence, one problem solved.
Then, I decided to do a preliminary trial to find the correction factor “f” as well as finding the surface tension of water at other different temperature instead of the room temperature. I used a “water drop counter” in this experiment, it will tell me precisely the volume of each water drop. However, there is a bit of a technical problem, since I’ve never used this kind of equipment before. Due to time constraint and trust issues, I decided to try to do the experiment manually. However, after I get the value for the surface tension of water at 60 Celsius and compare it to the literature value, I realise that the value I found is close to the real value but it’s also close to the other surface tension values of the other temperature (e.g. it’s also close to the value of the water at 40 Celsius), too. The error bar is too big that it overlaps with the value of the other independent variable.
Then, I realise that I shall change my equipment to more precision ones. That’s when I decided to measure the volume of the water drops using a precision mass balance to 3 decimal places and count the water drops to 50 instead. Then, I set up the whole experiment at the back of the science class so to prepare for the next time I came, so that I just have to conduct the experiment and record the results directly. 
Initial EE Reflection
I’m writing my EE in physics on the relationship between the temperature change and the surface tension of water. I plan to work out the value of the surface tension by using the Tate’s Law. What I found difficult is that the original Tate’s Law doesn’t have the variable (f) in its equation, however only later that this variable is introduced into the equation. Originally, this is a characteristic value given to the stalagmometer, an equipment used to measure the surface tension. However, I’m not using this equipment, I’m using a simple burette, and I need to find the value “f” for this. I can’t just search up the literature value for this since burettes are not usually used for this Tate’s equation. Hence, this has been stressful during the research process. So, my plan is to do more research on this, and if I am stuck, I shall approach my EE supervisor for advice and move on from that.